Distributed mass - dmass

The user needs only to specify the unitary mass (mass/length) value, from which the program computes internally the total element mass M, and subsequently derives the respective diagonal mass matrix with reference to the global translational degrees-of-freedom of the member.

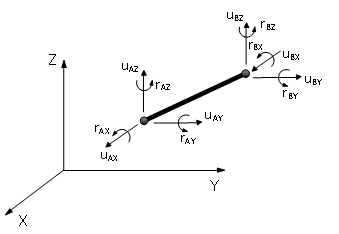
Notes

1. When the structure is subjected to very large deformations (e.g. buckling), the employment of two or more dmass elements per member is recommended, for accurate modelling.
2. If the loads are derived from masses (in the gravity direction based on the g value or in any translational direction, according to user-defined coefficients), then the program will automatically compute and apply ''distributed permanent loads'', herein effectively consisting of equivalent point forces/moments applied at the end nodes of the element (stress recovery will not have any effect in this case).
3. Distributed loads obtained from dmass elements are not considered in stress-recovery operations (because they are separate elements from the beams/columns), hence moment values throughout an element's length are bound to be wrong. Users interested in obtaining correct moments throughout an element's length, should define distributed mass/load using the 'material volumetric weight' in the Materials module and/or 'section added mass' in the Sections module.